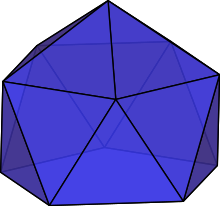
Gyroelongated pentagonal pyramid
| Gyroelongated pentagonal pyramid | |
|---|---|
 | |
| Type | Johnson J10 – J11 – J12 |
| Faces | 15 triangles 1 pentagon |
| Edges | 25 |
| Vertices | 11 |
| Vertex configuration | 5(33.5) 1+5(35) |
| Symmetry group | |
| Properties | convex |
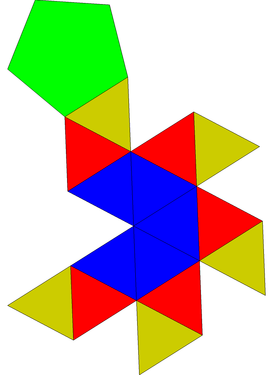
| Net | |
 | |

In geometry, the gyroelongated pentagonal pyramid is a polyhedron constructed by attaching a pentagonal antiprism to the base of a pentagonal pyramid. An alternative name is diminished icosahedron because it can be constructed by removing a pentagonal pyramid from a regular icosahedron.
Construction
[edit]The gyroelongated pentagonal pyramid can be constructed from a pentagonal antiprism by attaching a pentagonal pyramid onto its pentagonal face.[1] This pyramid covers the pentagonal faces, so the resulting polyhedron has 15 equilateral triangles and 1 regular pentagon as its faces.[2] Another way to construct it is started from the regular icosahedron by cutting off one of two pentagonal pyramids, a process known as diminishment; for this reason, it is also called the diminished icosahedron.[3] Because the resulting polyhedron has the property of convexity and its faces are regular polygons, the gyroelongated pentagonal pyramid is a Johnson solid, enumerated as the 11th Johnson solid .[4]
Properties
[edit]The surface area of a gyroelongated pentagonal pyramid can be obtained by summing the area of 15 equilateral triangles and 1 regular pentagon. Its volume can be ascertained either by slicing it off into both a pentagonal antiprism and a pentagonal pyramid, after which adding them up; or by subtracting the volume of a regular icosahedron to a pentagonal pyramid. With edge length , they are:[2]
It has the same three-dimensional symmetry group as the pentagonal pyramid: the cyclic group of order 10. Its dihedral angle can be obtained by involving the angle of a pentagonal antiprism and pentagonal pyramid: its dihedral angle between triangle-to-pentagon is the pentagonal antiprism's angle between that 100.8°, and its dihedral angle between triangle-to-triangle is the pentagonal pyramid's angle 138.2°.[5]
See also
[edit]References
[edit]- ^ Rajwade, A. R. (2001), Convex Polyhedra with Regularity Conditions and Hilbert's Third Problem, Texts and Readings in Mathematics, Hindustan Book Agency, pp. 84–89, doi:10.1007/978-93-86279-06-4, ISBN 978-93-86279-06-4.
- ^ a b Berman, Martin (1971), "Regular-faced convex polyhedra", Journal of the Franklin Institute, 291 (5): 329–352, doi:10.1016/0016-0032(71)90071-8, MR 0290245.
- ^ Hartshorne, Robin (2000), Geometry: Euclid and Beyond, Undergraduate Texts in Mathematics, Springer-Verlag, p. 457, ISBN 9780387986500.
- ^ Uehara, Ryuhei (2020), Introduction to Computational Origami: The World of New Computational Geometry, Springer, p. 62, doi:10.1007/978-981-15-4470-5, ISBN 978-981-15-4470-5, S2CID 220150682.
- ^ Johnson, Norman W. (1966), "Convex polyhedra with regular faces", Canadian Journal of Mathematics, 18: 169–200, doi:10.4153/cjm-1966-021-8, MR 0185507, Zbl 0132.14603; see table III, line 11.






